 use red/cyan anaglyph filters to see in 3D
use red/cyan anaglyph filters to see in 3D
 use red/cyan anaglyph filters to see in 3D
use red/cyan anaglyph filters to see in 3D
Stereoscopic images are taken from two camera viewpoints, separated horizontally, just as our eyes are separated horizontally (along the x axis). The distance between the two cameras is the stereoscopic base-line. In stricter terms, the distance between the first nodal points of the two lenses is the stereo base. Inter-ocular distance, iIt seems obvious that the correct distance between the two cameras will be the same as the separation between our eyes (correctly called the inter-ocular distance). Immediately there is a problem. People do not have the same inter-ocular distance. The audience for your 3D production will range from children, with close-set eyes to big-headed, large men. The inter-ocular distance (or inter pupillary distance) ranges through 48 - 73mm (adults) and 41 - 55mm (children). For manufacturing stereo microscopes, the range is often taken as 55 - 75mm The average adult inter-ocular distance is about 63mm for women and 65mm for men. 65mm has become the usual separation to aim for in stereoscopic photography. As you get more involved in stereoscopic photography, you will want to take magnified stereo of flowers or insects (macro 3D) or telephoto stereo of big wild animals or mountains (hyperstereo) and suddenly 65mm is not the best stereo base. Even more confusing, the stereo base is different depending on how you plan to view the images and a presentation made for a computer screen will not work on a movie theatre projection screen. |
The definition of stereo base is the distance between the first nodal points of the two lenses. This allows for a common method used in 3D cinema of converging the two cameras onto the object of interest. This convergence must occur around the first nodal points of the lenses, or the stereo base will change. The nodal point of a symmetrical magnifying glass is in the middle of the glass. Camera lenses have many glass elements spread along the barrel. The nodal point is split into a first node near the front of the lens and a second node near the back, or even outside the lens system. 3D movie-makers call the stereo base, the interaxial, (ia) This is fine, but is only true when the cameras are parallel, while in movies they often are not. Many even call the camera separation the interocular (io) and that is confusing, since cameras have lenses but only the audience have eyes.
|
|
You could purchase a stereo camera, or a beam splitter attachment. These only have one stereo base.
There is nothing to adjust - how boring.
In that case, this web site is not for you, being too technical for your needs.
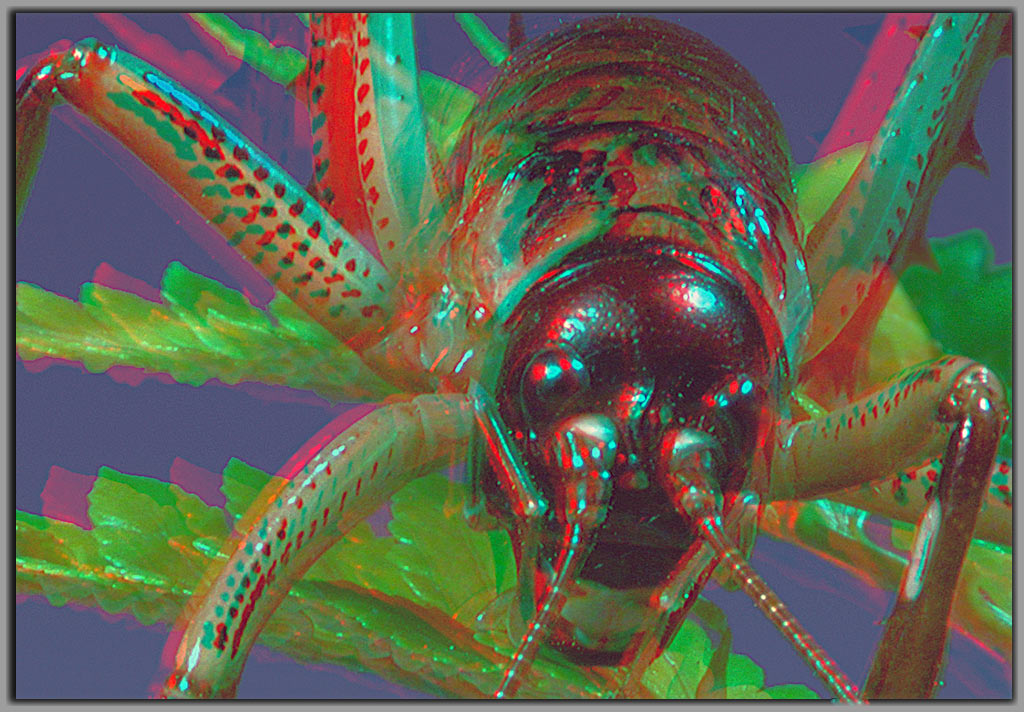
Weta
macroscopic stereoscopic anaglyph by John Wattie
Often you cannot achieve both acceptable disparity and perfect roundess.
In that case use the smaller of the two calculated bases, to avoid hurting anybody.
Anaglyphs are an easy way to understand stereoscopic disparity . Close objects (such as the debris covered glacier in the foreground) have no colour fringing. Close objects are superimposed on each other and have no disparity. They seem to be on the screen surface. This cannot be an orthostereoscopic image, since the glacier must be much further away than the screen surface, but we have learned to accept this 3 dimensional fiction on stereoscopic images. Especially in hyperstereoscopy, where the cameras are much further apart than our eyes are. The distant mountains are split into a cyan peak and a red peak. The further away objects are, the greater the disparity. The red mountains are to the left of the cyan mountains, which means this is uncrossed disparity. The left eye will see the red image and the right eye the cyan. The mountains are sitting in screen space.
Mt Cook and Mt Tasman, stereo photograph by John Wattie Maximum Disparity is the horizontal distance between the red and cyan images of the most distant mountain. On a portable computer the maximum disparity on New Zealand's second highest peak, Mt Tasman, is ~12mm, while on a 22 inch monitor it is ~15mm and 42 inch TV ~ 43mm (more if you enlarge the picture). That disparity is easily fused into 3D by everybody with normal vision who I have checked. Especially if they sit back. Few people want to see the big TV screen from less than 2 meters away. By sitting back, the disparity gets visually smaller, just as distant people look smaller than those nearby. Most people cannot diverge their eyes beyond parallel and so the maximum disparity, even for experts, should be less than their inter-ocular distance. But on a movie screen 20 meters away, disparity looks smaller and most people find more than 65mm separation can be fused at the movies, at least for a short time. Expert stereo observersYou might think Maximum Acceptable Disparity (MAD) would equal your interocular distance, because then your eyes are looking parallel, into the distance. Every time you drive a car, your eyes look into the far distance and they are parallel, so this happens every day for hours at a time. Experts in stereo viewing have no problem fusing 65mm disparity on a computer monitor. Naive observers, the changing focus problemUnfortunately most people cannot do that. As your eyes diverge to 65mm, they change focus automatically, because anything with that divergence must be a long distance off. But the 3D image is still close at hand, on the surface of the screen. Dilemma. Your eyes have to diverge, but then the nearby picture will be blurred. Oh no - a headache is coming on. When viewing deep stereoscopic images on a flat surface your eyes have to do two contradictory things: diverge to nearly parallel and focus only about 800mm away. Experts do that with no stress, but beginners are in trouble. Old people whose eyes can no longer focus have a rare advantage. Pop can put on his reading glasses to get a perfect image on the screen and then diverge into deep stereo without any problem, because his eyes cannot change focus anyway. Junior could probably get the same advantage by putting on 1.5 diopter reading glasses, under the anaglyph goggles. He then focuses on the screen 800mm away with his eyes focused for infinity by the magnifying spectacles. When he diverges into deep stereo, his eyes stay focused at infinity and cannot focus any deeper. Suddenly he has the same advantage as Grand-pop. I cannot prove this because my family refuse to put on two sets of glasses at once. Vanity is often a problem in the 3D world. Witness all the people who complain to me that 3DTV would be no good if they had to wear 3D goggles. Also they refuse to even put on anaglyph goggles, because they say they look stupid with two different coloured lenses and only geeks would bother with it. As for going cross-eyed to look at a picture - how silly can you get? Hopefully, if you are young and reading this, you are a "geek" who will try the experiment in the privacy of your own room? |
Stereoscopic rangeThe stereoscopic depth has to be coded in the acceptable disparity range, which is limited for an inexperienced audience. If there is a big distance between the nearest object and the furthest object, the disparity range available is not enough to show everything in full depth. The result is flat stereo or card-boarding. The disparity available to show 3D drops off by the square of the distance from the camera. Stereoscopic depth impression follows an inverse square law and falls off faster than perspective depth impression, which follows a linear relationship with distance from the camera. Better stereoscopic depth is obtained if the subject is not too thick, otherwise the impression of depth has to be compressed to fit into the acceptable range. The compression of stereoscopic depth is derived from the acceptable stereo base. A smaller base means more compression and less impression of depth. |
The stereoscopic pictures you produce have to be viewed in some device which presents the left eye picture to the left eye and right to right. There are many methods for seeing 3D, but there is one viewing parameter which is vital when setting the stereo base and that is the distance to the image, V. That is easy to measure for computer, TV and projection screens. Stereoscopes have a pair of lenses and the image is at a virtual distance, which varies with the focal length of the lenses and how they are focused. Most stereo viewers are set up so the virtual viewing distance is around about 2 meters. Television 3D is also usually set up for a 2 meter viewing distance. (A big TV screen in a pub, showing a sporting event, may well be seen from more than 2 meters and you just have to live with the stretched depth. Many people like big stereo depth, especially after a couple of beers). We have already seen how the stereoscopic viewing distance changes the shape of objects so that if you are too close they are squeezed and if you sit back they are stretched. You who are sitting in front of a computer monitor are potentially able to see a great 3D picture. But only if the stereo base was chosen to suit a person at 600 to 1000mm viewing distance. Mostly, stereo pictures are taken to suit a 2 meter viewing distance, or more commonly not properly set up at all, so do not feel too privileged in your computer chair. |


Glacial and River valleys, NZ by John Wattie. D = 3.0
Despite the considerable disparity, this has been a popular stereophotograph on Flickr, with 22 people marking it as a favourite and it shows up in stereo-photography image sets collected by enthusiasts.
If you are having difficulty, sit back.
Look at the forground first, then slide your gaze upwards into the distance.
This is using a "stereo ramp" to get your eyes adjusted to mildy deep stereo.
Stereoscopic roundness versus acceptable disparityStereoscopic roundness is the modern term for describing how deep objects look, also called stereoscopic perceived depth. The correct perception of roundness (perfect roundness, or roundness equals one) can be tested by photographing a sphere stereoscopically. Roundness is perfect when the diameter of the sphere is the same as the stereoscopically perceived depth of the sphere. Unfortunately roundness is not so simple to set up, because the perceived depth changes, depending on how far away the stereo pair are viewed from. Roundness for any particular viewing distance
So stereoscopic roundness is a useful concept which encapsulates all but one of the factors to be considered when setting the stereo base. Roundness does not include the factor of maximum acceptable disparity (MAD) which is measured by parallax (P). |
Two step process for setting stereo base
Acceptable disparity adjustment takes precedence over roundness, if you intend to show images to a naive audience. You see a lot of "flat stereo" at the 3D movies. Experts can fuse a much bigger disparity than neophytes. It is easier to set up good stereo depth for skilled viewers. Exactly how you achieve all this will be explained later. |
Stereoscopic Macro photographyThe stereo base for macro photography can be computed in various ways, but currently the author uses angular parallax for images larger than life size.
|