Contents for Stereoscopic Perceived Depth (Roundness)
Orthostereoscopy ][ Frame magnification ]
Conclusions and Comments on Roundness
Equations for perceived depth: S&S: 2
John Hart's equation for roundness
Contents for Stereoscopic Perceived Depth (Roundness)Orthostereoscopy ][ Frame magnification ] Conclusions and Comments on Roundness Equations for perceived depth: S&S: 2 John Hart's equation for roundness |
If you want a perfect 3D experience, use a dedicated stereo camera
and view the results in a matched stereoscope.
On the computer, real size and depth is a hassle.
On a big projection screen, real size and depth is nearly impossible.
Don't worry about orthostereoscopy, as it is not essential for a happy 3D experience,
but the geometrical concepts involved teach us a lot about 3D photography.
Stereo on a computer can be made orthoplastic. But hardly anybody bothers about orthostereoscopic, except fanatics like me and I only do it on rare occasions, for fun. Dedicated stereo cameras produce orthostereoscopic scenes, if they are viewed in a suitable stereoscope:
When the image is viewed on a computer, it is difficult to get an orthostereoscopic image. Usually the photographer gives up and concentrates instead on making a satisfying depth impression in his picture, not caring too much if it reproduces reality or not. Orthoplastic is nice, but not essential.
Makers of binocular head mounted displays (HMD) need the graphics to match the real world and for them orthostereoscopy is an essential consideration. They must consider field of view of the display, varying inter-ocular distance of the observers and magnification, just as photographers must. In addition there are distortions from the convex lenses which are required to make the image seem to sit at the correct distance and in focus. Usually the true image is very small in an HMD and strong magnification is needed, plus a wide image field (typically 40 degrees). The image needs to be distorted to conteract the geometric distortion of the lenses. |
|
Orthostereoscopic when viewed from 1080mmThe anaglyph below is as close as I can get to orthostereoscopic, but only at 1080mm viewing distance from the computer screen. Distance to the nearest object during photography was 1080mm. If your eyes are not 1080mm away from your computer monitor, the image cannot be orthostereoscopic.
Stereo base (distance between the two cameras) was 65mm, which is the average human inter-ocular distance. Already this picture is not quite orthostereoscopic for my 68mm eye separation. Magnification of the foreground is one, but only on my 22 inch computer screen running at 1680x1050 pixels. The aneroid altimeter is 80 mm diameter. Since perspective makes it look oval when viewed without the anaglyph glasses, check the maximum, transverse diameter. If it is not 80mm, there is a magnification problem with orthostereoscopy on your computer. Only an object in the stereo window, at the level of the screen, is going to have normal size (magnification of 1). Objects further back must be smaller, by perspective. In an orthostereoscopic image, perspective size reduction and stereoscopic depth fade out with distance, while keeping "in normal step" with each other. Perspective makes size reduce in proportion to distance. Stereoscopic depth reduces in proportion to the square of the distance. So keeping 3D and perspective in "normal step" is a bit of a mathematical conundrum, which our brains are able to handle subconsciously, if they have been trained since birth with normal stereoscopic vision. But our conscious thinking of linear versus inverse square, dating from high school maths, often becomes confused!
D = 1 |
|
 |
Orthostereoscopic from 2 meters
Notice how the perspective is different from the first image of the chess board. Front squares are closer and so look bigger than distant squares, but the size difference from front to back is less in this 2 meter image than on the 1.08 meter picture. The difference in perspective of the squares and roundness of the barometer can be seen, if you are critical, even without putting on the anaglyph goggles. But in 3D the difference is obvious, while in 2D you have to think about it. When viewed from less than 2 meters, the stereo depth (roundness) is too flat and the perspective is too small. Only at 2 meters are roundness and perspective both correct - if your eyes are 65mm apart.
D = 1 |
|
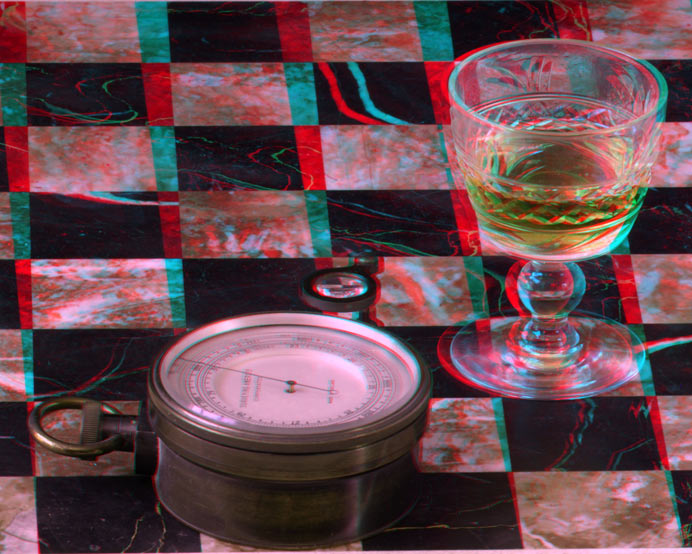 |
Anaglyph: use red/cyan goggles to see in 3D
Difficulty viewing index: D = 6.5
Already there is a problem with orthostereoscopic pictures which include distant objects. Only experts at viewing stereo pairs can actually fuse them! This is unexpected since we are all used to looking through windows. As explained before, the problem is uncoupling the focus of your eyes from the convergence of the two eyes. This is a learned skill, essential for a 3D image, which actually lies on a flat surface and only gives the illusion of depth.

The aim was to turn the computer screen into a "window on the world." Photographing through an actual window to set up this orthostereoscopic concept of virtual reality was suggested to me by Jay Gharavi of Ventura, California (jaysdesk). The problem was finding a small enough window. The stereo pair were photographed 1 meter away from the window, with a mildly wide-angle lens: 35mm focal length in 35mm equivalent camera terms. The digital camera was far smaller than full frame 35mm, which is how the picture has such a huge depth of field. The stereo base between the two cameras was 65mm, which is the average inter-ocular distance for a male adult. The window and beyond is orthostereoscopic only when viewed from 1 meter. The image magnification has been set to get the window at natural size on my computer screen, but if you do not have the same size pixels on your screen, it will not be quite right. Even on my 22 inch monitor, the window is too big to fit and I have to pan around slightly. The disparity of the most distant points in the stereoscopic scene has to be 65mm (this distant part is nearly lost in the glare of the sky around the setting sun, top right.) Disparity can be measured on an anaglyph as the distance between the red and cyan images of the same point (homologous points). Objects at infinity on an orthostereoscopic picture are separated by 65mm
|
Howick Historical Village. The window is in a restored, 19th century, tiny, wooden, school house. (Dame School.) The glass is old and not perfectly flat. It acts like a wavy lens, distorting the distant building, which is a blacksmith's shop. The 2 left panels are not distorting and must have been replaced with modern glass.When I was a child, all house windows distorted like this and plate glass was only seen in shop windows or other expensive installations. The distortion is different for each eye, since they are looking through different parts of the rippled glass, and that will add to the difficulty of fusing the image. Experienced stereoscopists may not have difficulty fusing mild distortion like this, but even an experienced worker (Louis Carlsson) protested about this picture. That is why orthostereoscopy is not worth the effort, except for your private use. |
The dynamic range is very high, looking from a dark room into the bright sky around the setting sun. Usually that is impossible for anaglyph manufacture, or even for getting a photograph at all. The secret is HDR: High Dynamic Range digital photography. Three pictures are taken with 2 stops difference in exposure between them:
They are then fused in a computer program, in this case Photomatix. The image you see comes from 6 separate exposures (3, plus another 3 for the other eye.) |
Big screenOn a computer screen, we can only see small, close objects orthostereoscopically. But big movie screens also have problems in showing a realistic "window on the world." The above images projected onto a large screen would not be orthostereoscopic, unless the magnification was zoomed out until the barometer was 80mm across and you stood 1 meter from the screen. That would be impossible, unless you owned the movie theatre. Roundness at the movies can be orthoplastic but not orthostereoscopic. Orthostereoscopy on a movie screen, 20 meters away, would be great for showing a large hall at full size. Scenery could be orthostereoscopic, as long as the nearest object in the scene was exactly 20 meters away, the camera lenses had the same angle of coverage as the screen subtends 20 meters away and a 65mm stereo base-line was used. The furthest object would need to be aligned on the screen with a 65mm or less deviation between homologous infinity points. So, you are not going to see orthostereo at the movies, even if you sit in the one seat the 3D image has been set up for. That seat is called the ortho-stereoscopic seat. Most movies are not shot for orthostereoscopy - why bother when only one member of the audience can see it that way? Even worse, when you use a 65mm base on movie cameras, the stereo disparity for distant objects on a big movie screen becomes so big the audience has to diverge their eyes. That is painful or impossible for most people and is forbidden in modern 3D movies. Excess disparity can be overcome by bringing the stereo window forwards, off the screen, into the movie theatre (floating window technique), but then orthostereoscopy is definitely lost. The best seat in the movies is certainly in the centre line of the screen, but how far back depends on how the movie was photographed and may even change during the program. Do not sit close to the screen: the 3D will be flat and the deviation of distant objects may well be uncomfortable. Sit centrally, at the back and you will see the 3D well, but it may well be exaggerated, with excessive depth. Many people prefer too much depth, saying there is little point paying extra for 3D movies unless you see lots of depth. Stereoscopic projection is too dim. There is a set of filters on the projector and another set worn by the audience, both reducing light. The light is "chopped up" as the frames change, introducing a black phase, which reduces over-all brightness. Modern 3D movies are going to very high frame rates to ensure movement is smooth and there is no flicker, so the duty cycle will always be reduced. Lower brightness means lower resolution and dimmer colours. The colour shifts from normal reds to dominant blues (Purkinje shift). Brighter lights to overcome this mean more heat and burnt-out bulbs. 2D movies can run at 48 candelas per meter squared but 3D movies drop to 10c/m2 behind the glasses. Lasers would be good in the projectors, but there is no white light laser yet. The screen has to be metallic, or the polarisation will be scrambled. A metallic screen introduces a central hot spot. People in the center line get a bright image, those at the sides of the theatre see a dimmer version and the deterioration begins just 3 seats from the centre line. Dolby and Xpan D systems do not need a silver screen and the sweet spot problem is reduced. |
Orthostereoscopy seat: formulaV = MF V = the correct Viewing distance to sit from the screen (projection, TV or computer) to see an orthostereoscopic image.
MagnificationFrame magnification(Width of the screen) / (Width of the frame) or, same thing:- (screen height) / (frame height) This definition comes from photography, where the frame is the film frame, or the size of the gate in the camera. A 35mm film frame is 24 x 36mm.
Object magnification(Object size in the final image) / (Real size of the object) The aneroid barometer in the above images has an object magnification of one, at least it does on my computer screen. The real diameter and the image diameter are both 80mm. Object magnification =
35mm correction factorFor 35mm "full size sensor" digital cameras, the 35mm correction factor is 1. For 4/3 format digital cameras (Olympus, Panasonic) the sensor is half the 35mm size and the 35mm correction factor is 2. Small digital cameras have all sorts of 35mm correction factors, but the factor is quoted in the instruction manual. The true lens focal lengths are also in the manual, but are usually forgotten because they are only referred to as their 35mm equivalents. When you buy the camera you only hear about 35mm equivalent lenses in the shop. Macro lensA macro lens can have its magnification set from a scale on the lens as you wind it out. This is magnification in the camera, not the final magnification you see on the computer or a paper print. At a magnification of 1, the image on the sensor is the same size as the object. If you are using a small sensor, only part of that image fits on the sensor, as compared with a "full frame camera." So, if 1:1 magnification is set on the macro lens, In the 4/3 system (Olympus, Panasonic), where the sensor is half size, magnification with a macro lens is twice that of a true 35mm camera, once the image is set up on your computer for viewing. This has important consequences in digital macro stereo-photography. When you are on this web site, only enter 35mm equivalent focal lengths into the equations.Aspect ratio
Stereoscopic images are often cropped from the sides in order to set the stereo window, and the only "constant" becomes the frame height. Perspective invarianceFor this web site, I have chosen the 35mm standard frame size for all mathematical formulae. Digital cameras usually have smaller frames, but they give the same perspective and stereo disparity as a 35mm camera, if the tiny image sensor is multiplied by a ratio to bring it up to 24mm vertical height. This is allowable because cameras have a property called perspective invariance. That means perspective does not change with different size cameras, as long as the angle of view in the cameras is the same. If a camera size and its lens focal length are scaled up or down to match the size of a 35mm film frame it is called 35mm equivalent. All digital cameras you buy are quoted in terms of 35mm equivalent. They will give enlarged images (say post-card size, for example) with the same perspective as the equivalent 35mm camera does. The images are often better, because small cameras have a bigger depth of field than 35mm cameras and the post-cards produced look sharper. But the perspective on the post cards looks the same as from a 35mm film camera, by perspective invariance. |
It is possible to arrange correct roundness for any one particular viewing distance,
but not all and perspective will be wrong, unless the principles of orthostereoscopy are followed.
A mismatch between perspective and roundness causes distortion of 3D perception.
Minification, Giantism, Puppet theatre effect, Frustum distortion, Card-boarding.
Experienced 3D people learn to ignore the perspective/roundness mismatch,
but folk new to 3D are usually upset by it.
If you want a perfect 3D experience, only use a dedicated stereo camera
and only view the results in a matched stereoscope.