Stereoscopic Roundness
depends on Four Parameters

Roundness in 3D is very sensitive to how far you are from the viewing screen.

Try moving backwards and forwards, wearing your anaglyph goggles, until the sphere is spherical, just like a ball .

A sphere should appear at 1000mm from the screen, if your eyes are 65mm apart, which is average.

The grid has 10mm squares. If they are 10mm on your screen, then the scene is orthosteroscopic. That means the stereo anaglyph is as close as possible to reality.

You may need a big computer screen to see this ortho-stereo

Undistorted viewing

True roundness does not need to be orthostereoscopic. As long as the ball looks round, it is not distorted and its size is not important.

Once the stereo pair have been photographed, roundness persists at any magnification, but only if you keep viewing from the same distance of 1 meter .

Roundness in 3D is very sensitive to how far the camera was from the ball and the stereo base

1. Distance to the background grid: 1000mm
2. Stereo Base: 65mm
3. B/n = 0.065 = aprox 1/15
4. angular parallax = 3.7 degrees.

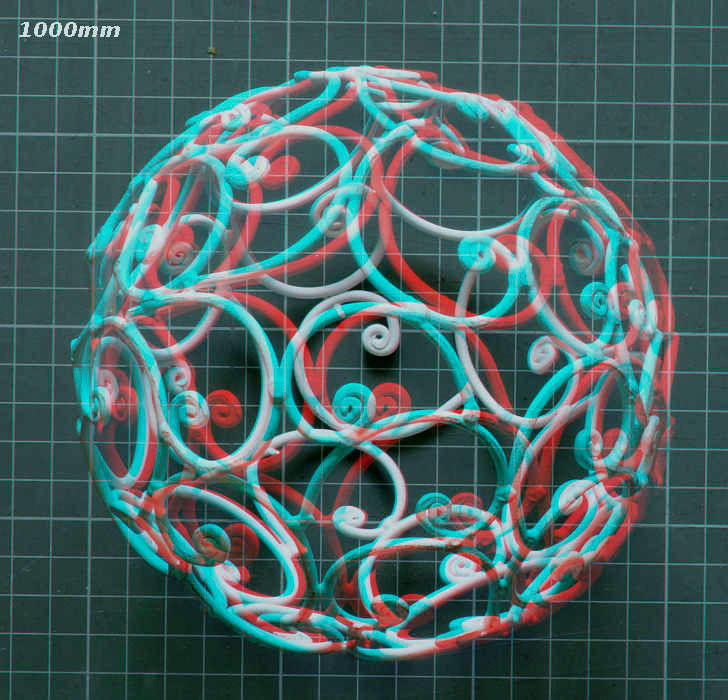
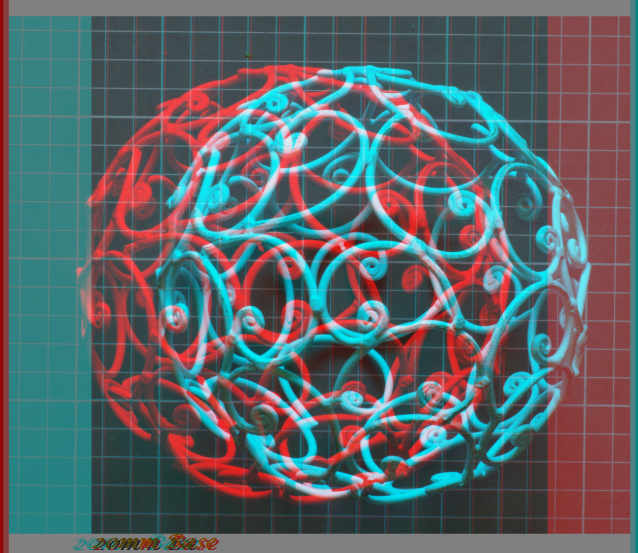
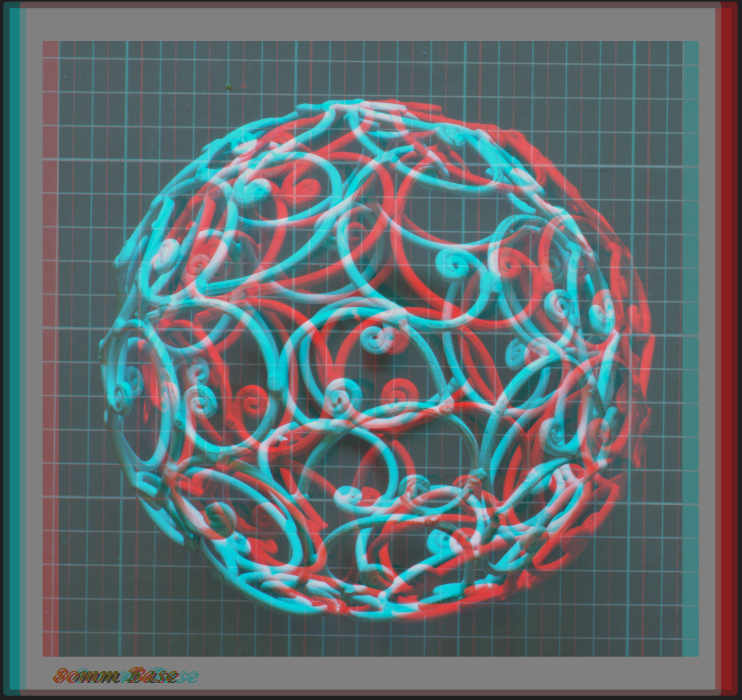
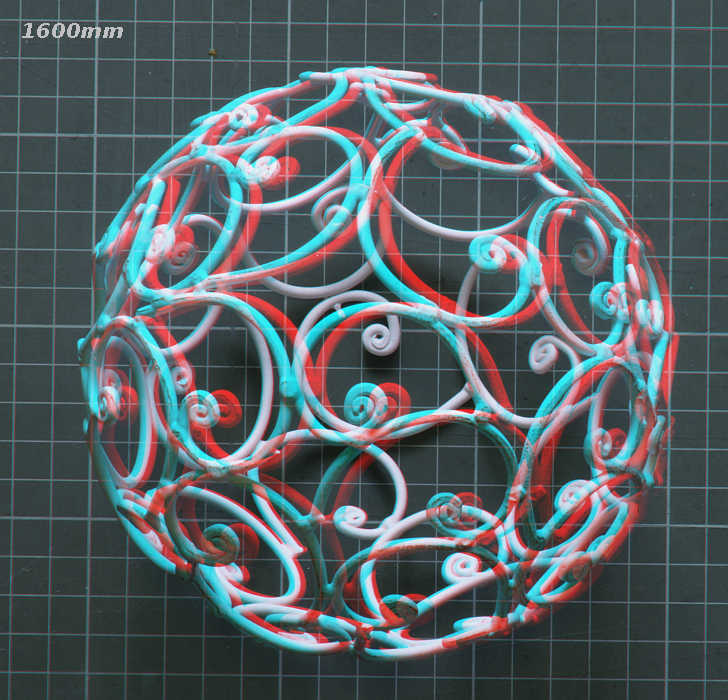
Flattened Sphere

You are probably still looking at the screen from 1 meter but the sphere now seems deflated.

Move back until it is a ball again and you will be sitting at near enough to 1600 mm from the screen.

The magnification is the same as the 1000mm view example. Check by measuring the grid.

Now the scene is not orthostereoscopic. The cameras have not reproduced the distance between your eyes, but are closer together, at 40mm, than even a child's eyes.

You have to sit 1.625 times further away (65/40) .

Stretch

By moving back you have stretched the sphere in the depth plane (z) to make it round.

Squeeze

Sitting closer, at a more usual distance, the sphere is flattened.

Photography factors

1. Distance to the grid 1 meter
2. Stereo Base 40mm
3. B/n = 1/25 = 0.04
4. angular parallax = 2.29 degrees

Persistence of perspective and

Persistence of perceived stereo depth,

Despite a change in magnification.

The experiment shows roundness, like perspective, is not changed by magnification of the image.

M = v/u

M: Magnification on the receptor (film or CCD)
v: Distance of the lens from the receptor
u: Distance of the lens from the subject.

v = F + E

F : Focal length of the lens
E: Extension, or the extra distance the lens moves from its infinity position to focus the subject.
n: Distance to nearest object, which is important in stereo photography.

M = (F + E) / n

(Only true if the nearest object has been focused on, but works pretty well in macro photography, which is the situation you really care about magnification.)

Final magnification on your computer screen depends on how much the receptor image is further magnified, (frame magnification) which gets complex if the image is both cropped and resized.

This Column For Specialists. Avoid on your first reading:

Relative Parallax Invariance with Magnification

No matter what magnification you view the sphere at, perfect roundness persists, as long as your distance to the screen (V) does not change.

If you zoom into a stereo picture while viewing it (as you can easily do in a program like StereoPhotoMaker) roundness does not vary. Just as roundness has not varied here with magnification change.

Perspective Invariance

Perspective behaves the same way. The relative magnification of objects to each other at different distances in the scene does not change when you magnify the whole picture. A chair half the height of a table stays half the height as the image size changes. That is why a tiny digital camera can take a picture just as realistic as a large view camera photographing from the same position. Except the small camera result is more noisy, less sharp and shows a bigger depth of field.

Magnification in photography is determined by focal length of the camera lens (combined with the extension needed to focus it), plus any change in picture size made during post-processing of the image. (Photographic magnification multiplied by frame magnification).

The experiment shows roundness, like perspective, is not changed by magnification. That is the same as saying the focal length of the camera lens has no effect on these two indicators of depth. But it only works as long as the distance from camera to nearest object, n, is kept constant as the lens is zoomed.

You need to ponder that because it is counter-intuitive. We are used to thinking that a telephoto lens flattens perspective. But that is only because you zoom in to magnify distant objects, which means you are photographing from further away than usual (n is bigger).

We say that telephoto stereo causes card-boarding (which is the same as saying roundness has decreased). Again that happens because you usually take telephoto shots from further away (n has increased).

If you use telephoto from further away, you must increase the stereo base. Twice as far away needs twice as much base to retain roundness (or no card boarding). BUT beware, because increasing the base can cause excessive stereo deviation, as we will see later.

Football Code Deformity

If you are back at 2 meters, the sphere has become an ellipsoid, like an egg pointing at you.

A soccer ball changes code to become a rugby ball.

Getting it back to spherical means moving closer, to just over 800mm away from the screen.

Base over nearest object.

B / n

Here B/n is 1/12.5

B/n is the rule you can use while taking a photograph to get a perfect shape in a stereo picture, but it is not a constant because it must be set to equal:

Interocular over screen distance

i/V

Here i/V is 65/813 = 1/12 .5

But only if your interocular distance is 65mm.

My interocular distance is 68mm, so for perfect roundness I need to sit at
68N/B = 68000/80 = 850mm

Photography factors

1. Stereo Base 80mm
2. Distance to the grid 1 meter
3. B/n = 1/12.5 = 0.08
4. Angular parallax = 4.58 degrees

Correction attempt with floating window

Instead of the observer moving back, the stereoscopic image itself can be moved further away with floating windows.

Photographic factors

1. Base 20mm
2. Distance to grid 1000mm
3. B/n = 1/50 = 0.02
4. Angular parallax = 1.15 degrees
   

To my eye, the floating window experiment has failed to correct the lack of roundness.

However, the ball looks bigger because I think it is further away, where it should look smaller.

In truth, the image of the ball has not changed size and it is still sitting on the flat screen.

Perceptually, the ball has retreated behind the screen and now looks bigger, because we think it is further away. But it has stayed just as flat as ever and measurement reveals it has not changed size.

This is a good example of a stereoscopic illusion. It shows our perception of depth does not follow Euclid's laws of geometry. Depth perception is a learned experience and since it is an illusion, it is hard to measure.

So how about trying to make the roundness worse by floating a sphere, good for viewing close up on the screen, forwards to where it might flatten?

The sphere is correct for 813 mm view distance, an average sort of computer screen viewing distance

Floating the sphere forward should make the spherical deformity worse but to my eye it is no different. The back of the sphere has moved forwards, balanced by the same for the front of the sphere.

Moving the stereo window forward or back does not help or hinder roundness.

There seemed to be a pattern to the viewing distance required to make spheres look round. As a guess I thought the viewing geometry should be in proportion to the taking geometry.

I decided to compare human measurements with conceptually similar camera measurements, by ratios.

By rearranging the ratios, I attempted to predict the correct viewing distance from the camera geometry, by making the ratios equal.

V/n = i/B

V: Viewing Distance

n: Lens distance to the grid

i: Interocular distance: taken to be the human average of 65mm

B: Stereo Base

The viewing distances, V, predicted from this formula for correct roundness are shown on the top left of each image.

Rearranging the ratios:

V= i n / B

= 65N/B

Now anybody can check how far their eyes should be from the screen (V) to get the impression of a round sphere. The distances marked on the top left should be correct, if your eyes are 65mm apart.

If your interocular distance (i) is much different from 65mm, it is necessary to run the formula to see if that gives you a better personal viewing distance.

This experiment did not measure stereoscopic roundness, it is based on a binocular visual assessment.

Olivier Cohen has since told me this was all done last century. I am delighted. That is not really the point since this is a learning web site, not a scientific paper and I do not claim to have discovered anything! For historic interest, I would love to know who did it though.

Geometry of perceived depth

Subsequently, I sorted out the mathematics for perceived depth and found everything stated here, based on perception, works out in formal geometry too. I can measure depth now. Geometry explains the way magnification (zooming) makes no difference to perceived depth and the way objects are stretched and squeezed as the viewing distance changes. See stretch and squeeze 2.  I bet somebody else has worked all this out, I just cannot find where he published it!

Formula for an undistorted viewing distance, V

(Viewing distance) = (Interocular) * n / (Stereo Base)

Viewing distance is the distance from your eyes to the screen.
n is the distance in mm from the camera lens' first nodal point to the object which is to end up on the screen surface. This is frequently the distance from the camera to the nearest object, because when the stereo window is set during post-processing, the nearest object is arranged to be on the screen surface. In this experiment, the grid ended up on the screen and so n is the distance to the grid.
Interocular distance is the distance between the observer's eyes. Obviously children and women have closer set eyes than large men, but the adult average is around 65mm.
Stereo Base is the distance between the lenses when the picture was taken (mm). (Actually, measure from first nodal point of the lenses, to allow for any toe-in) .

Formula for stereo base, B, to produce undistorted pictures.

Re-arranging the ratios to give Base.

(Base) = (Nearest object) * (Interocular distance) / (Viewing distance)

B = n i / V

This means that, while you are taking the stereo pair and aiming for perfect shape depiction (orthoplastic), the distance between the two cameras (stereo base) has to be set up differently, depending on how you plan to view the pictures.

Formula for degree of roundness, R

Stereo pictures are often not taken with the stereo base required for correct roundness (roundness = 1) on the viewing screen actually being used.

"Degree of roundness" is not a constant value for any stereo pair of pictures:

1. Our experiment shows roundness changes as the viewing distance, V, changes.
2. Roundness is also different for different observers, which is neglected when inter-ocular distance, i, is taken as 65mm for everybody.

Roundness can be measured in terms of the stereo base used.

Roundness = [Stereo base actually used] / [Base computed for perfect roundness]

Roundness = [Stereo Base Used] * V / n i

Or roundness can be computed in terms of viewing distance:

R = [Viewing distance actually used] /
      [Viewing distance set for the  orthoplastic stereo picture]

A picture with a roundness of 1 on a 3DTV screen, viewed from 2 meters;
will drop to 0.5 on a computer screen, viewed from 1 meter .

Examples

I have a Zalman 3D computer screen and I like to view it from 1 meter (V), because closer than that the interlace lines become too obvious.
A typical closest distance in stereo photography (n) is 2 meters (6 feet).

Nearest Object: 2000mm
Interocular (average human): 65mm
Viewing distance: 1000mm

Base = n i / V = 2000 * 65 / 1000 = 130mm

Base / Nearest = 130 / 2000 = 1/15

This result is more interesting than you may realise.

Digital cameras set up side by side cannot get the lenses closer than about 130 to 140mm. Some experts say this is no good for 3D, because the cameras are too far apart. Ah, but, they have not thought about roundness and viewing the results on a computer monitor. It turns out the 135mm stereo base for a pair of Sony V3 cameras is just right for computer stereo.

BUT the stereo pairs for a computer or book cannot be projected without causing too much stereo deviation. (Maximum Acceptable Deviation, MAD, is exceeded.)

SO computer users and projectionists end up having a fight!

What is the roundness if we view a 3D picture, designed for computer viewing, on a 3D television 2 meters away?

New V = 2000mm
Used B = 130mm

Roundness = [Stereo Base Used] * V / n i

Roundness = 130 * 2000 / (2000 * 65 )
Roundness = 2

So; things looking perfectly round on a computer monitor, or when printed in a book, look twice as round as they should on a 3D television 2 meters away. If the TV is viewed from 3 meters, the roundness is 3, which is beginning to look ridiculous.

There is a rule in photography, which some people are madly insistent on, called the 1/30 rule for setting stereo base

(B/n = 1/30)

OH NO it seems 1/30 rule is going to give you flat pictures, if they are to be seen on a computer screen!

On a movie screen the 3D depth will be really excessive, if the 1/30 rule is followed. So the 1/30 rule is not always correct.

The 1/15 rule for macro photography was derived in a very different way by Frank Di Marzio in the first edition of this web site. It was based on MAD and not roundness considerations.

Now I want to view a 3D picture of my family on a television 2 meters away, instead of on my computer. The stereo base required to get perfect roundness is smaller. In fact it is exactly the same as the viewer's interocular distance.

n: 2000
i : 65
V: 2000

Base = n i / V = 2000 * 65 / 2000 = 65mm

B/n = 1/30

So the 1/30 rule is correct if you are viewing the stereo image from 2 meters - which was a very common distance before computers.

Using a lens stereoscope, such as a Holmes viewer, people adjust the virtual optical distance to the stereo pair by changing the focus. They usually end up at a virtual viewing distance of around 2 meters, so the 1/30 rule has a wide application.